The Elegant Universe: Superstrings, Hidden Dimensions, and the Quest for the Ultimate Theory - Brian Greene (2010)
Part II. The Dilemma of Space, Time, and the Quanta
Chapter 5. The Need for a New Theory: General Relativity vs. Quantum Mechanics
Our understanding of the physical universe has deepened profoundly during the past century. The theoretical tools of quantum mechanics and general relativity allow us to understand and make testable predictions about physical happenings from the atomic and subatomic realms all the way through phenomena occurring on the scales of galaxies, clusters of galaxies, and beyond to the structure of the whole universe itself. This is a monumental achievement. It is truly inspiring that beings confined to one planet orbiting a run-of-the-mill star in the far edges of a fairly ordinary galaxy have been able, through thought and experiment, to ascertain and comprehend some of the most mysterious characteristics of the physical universe. Nevertheless, physicists by their nature will not be satisfied until they feel that the deepest and most fundamental understanding of the universe has been unveiled. This is what Stephen Hawking has alluded to as a first step toward knowing "the mind of God."1
There is ample evidence that quantum mechanics and general relativity do not provide this deepest level of understanding. Since their usual domains of applicability are so different, most situations require the use of quantum mechanics or general relativity, but not both. Under certain extreme conditions, however, where things are very massive and very small—near the central point of black holes or the whole universe at the moment of the big bang, to name two examples—we require both general relativity and quantum mechanics for proper understanding. But like the mixing of fire and gunpowder, when we try to combine quantum mechanics and general relativity, their union brings violent catastrophe. Well-formulated physical problems elicit nonsensical answers when the equations of both these theories are commingled. The nonsense often takes the form of a prediction that the quantum-mechanical probability for some process is not 20 percent or 73 percent or 91 percent but infinity. What in the world does a probability greater than one mean, let alone one that is infinite? We are forced to conclude that there is something seriously wrong. By closely examining the basic properties of general relativity and quantum mechanics, we can identify what that something is.
The Heart of Quantum Mechanics
When Heisenberg discovered the uncertainty principle, physics turned a sharp corner, never to retrace its steps. Probabilities, wave functions, interference, and quanta all involve radically new ways of seeing reality. Nevertheless, a die-hard "classical" physicist might still have hung on to a thread of hope that when all was said and done these departures would add up to a framework not too distant from old ways of thinking. But the uncertainty principle cleanly and definitively undercut any attempt to cling to the past.
The uncertainty principle tells us that the universe is a frenetic place when examined on smaller and smaller distances and shorter and shorter time scales. We saw some evidence of this in our attempt, described in the preceding chapter, to pinpoint the location of elementary particles such as electrons: By shining light of ever higher frequency on electrons, we measure their position with ever greater precision, but at a cost, since our observations become ever more disruptive. High-frequency photons have a lot of energy and therefore give the electrons a sharp "kick," significantly changing their velocities. Like the frenzy in a room full of children all of whose momentary positions you know with great accuracy but over whose velocities—the speeds and directions in which they are moving—you have almost no control, this inability to know both the positions and velocities of elementary particles implies that the microscopic realm is intrinsically turbulent.
Although this example conveys the basic relationship between uncertainty and frenzy, it actually reveals only part of the story. It might lead you to think, for instance, that uncertainty arises only when we clumsy observers of nature stumble onto the scene. This is not true. The example of an electron violently reacting to being confined in a small box by rattling around at high speed takes us a bit closer to the truth. Even without "direct hits" from an experimenter's disruptive photon, the electron's velocity severely and unpredictably changes from one moment to the next. But even this example does not fully reveal the stunning microscopic features of nature entailed by Heisenberg's discovery. Even in the most quiescent setting imaginable, such as an empty region of space, the uncertainty principle tells us that from a microscopic vantage point there is a tremendous amount of activity. And this activity gets increasingly agitated on ever smaller distance and time scales.
Quantum accounting is essential to understand this. We saw in the preceding chapter that just as you might temporarily borrow money to overcome an important financial obstacle, a particle such as an electron can temporarily borrow energy to overcome a literal physical barrier. This is true. But quantum mechanics forces us to take the analogy one important step further. Imagine someone who is a compulsive borrower and goes from friend to friend asking for money. The shorter the time for which a friend can lend him money, the larger the loan he seeks. Borrow and return, borrow and return—over and over again with unflagging intensity he takes in money only to give it back in short order. Like stock prices on a wild, roller-coaster day on Wall Street, the amount of money the compulsive borrower possesses at any given moment goes through extreme fluctuations, but when all is said and done, an accounting of his finances shows that he is no better off than when he began.
Heisenberg's uncertainty principle asserts that a similar frantic shifting back and forth of energy and momentum is occurring perpetually in the universe on microscopic distance and time intervals. Even in an empty region of space—inside an empty box, for example—the uncertainty principle says that the energy and momentum are uncertain: They fluctuate between extremes that get larger as the size of the box and the time scale over which it is examined get smaller and smaller. It's as if the region of space inside the box is a compulsive "borrower" of energy and momentum, constantly extracting "loans" from the universe and subsequently "paying" them back. But what participates in these exchanges in, for instance, a quiet empty region of space? Everything. Literally. Energy (and momentum as well) is the ultimate convertible currency. E = mc2 tells us that energy can be turned into matter and vice versa. Thus if an energy fluctuation is big enough it can momentarily cause, for instance, an electron and its antimatter companion the positron to erupt into existence, even if the region was initially empty! Since this energy must be quickly repaid, these particles will annihilate one another after an instant, relinquishing the energy borrowed in their creation. And the same is true for all of the other forms that energy and momentum can take—other particle eruptions and annihilations, wild electromagnetic-field oscillations, weak and strong force-field fluctuations—quantum-mechanical uncertainty tells us the universe is a teeming, chaotic, frenzied arena on microscopic scales. As Feynman once jested, "Created and annihilated, created and annihilated—what a waste of time."2 Since the borrowing and repaying on average cancel each other out, an empty region of space looks calm and placid when examined with all but microscopic precision. The uncertainty principle, however, reveals that macroscopic averaging obscures a wealth of microscopic activity.3 As we will see shortly, this frenzy is the obstacle to merging general relativity and quantum mechanics.
Quantum Field Theory
Over the course of the 1930s and 1940s theoretical physicists, led by the likes of Paul Dirac, Wolfgang Pauli, Julian Schwinger, Freeman Dyson, Sin-Itiro Tomonaga, and Feynman, to name a few, struggled relentlessly to find a mathematical formalism capable of dealing with this microscopic obstreperousness. They found that Schrödinger's quantum wave equation (mentioned in Chapter 4) was actually only an approximate description of microscopic physics—an approximation that works extremely well when one does not probe too deeply into the microscopic frenzy (either experimentally or theoretically), but that certainly fails if one does.
The central piece of physics that Schrödinger ignored in his formulation of quantum mechanics is special relativity. In fact, Schrödinger did try to incorporate special relativity initially, but the quantum equation to which this led him made predictions that proved to be at odds with experimental measurements of hydrogen. This inspired Schrödinger to adopt the time-honored tradition in physics of divide and conquer: Rather than trying, through one leap, to incorporate all we know about the physical universe in developing a new theory, it is often far more profitable to take many small steps that sequentially include the newest discoveries from the forefront of research. Schrödinger sought and found a mathematical framework encompassing the experimentally discovered wave-particle duality, but he did not, at that early stage of understanding, incorporate special relativity.4
But physicists soon realized that special relativity was central to a proper quantum-mechanical framework. This is because the microscopic frenzy requires that we recognize that energy can manifest itself in a huge variety of ways—a notion that comes from the special relativistic declaration E = mc2. By ignoring special relativity, Schrödinger's approach ignored the malleability of matter, energy, and motion.
Physicists focused their initial pathbreaking efforts to merge special relativity with quantum concepts on the electromagnetic force and its interactions with matter. Through a series of inspirational developments, they created quantum electrodynamics. This is an example of what has come to be called a relativistic quantum field theory, or a quantum field theory, for short. It's quantum because all of the probabilistic and uncertainty issues are incorporated from the outset; it's a field theory because it merges the quantum principles into the previous classical notion of a force field—in this case, Maxwell's electromagnetic field. And finally, it's relativistic because special relativity is also incorporated from the outset. (If you'd like a visual metaphor for a quantum field, you can pretty much invoke the image of a classical field—say, as an ocean of invisible field lines permeating space—but you should refine this image in two ways. First, you should envision a quantum field as composed of particulate ingredients, such as photons for the electromagnetic field. Second, you should imagine energy, in the form of particles' masses and their motion, endlessly shifting back and forth from one quantum field to another as they continually vibrate through space and time.)
Quantum electrodynamics is arguably the most precise theory of natural phenomena ever advanced. An illustration of its precision can be found in the work of Toichiro Kinoshita, a particle physicist from Cornell University, who has, over the last 30 years, painstakingly used quantum electrodynamics to calculate certain detailed properties of electrons. Kinoshita's calculations fill thousands of pages and have ultimately required the most powerful computers in the world to complete. But the effort has been well worth it: the calculations yield predictions about electrons that have been experimentally verified to an accuracy of better than one part in a billion. This is an absolutely astonishing agreement between abstract theoretical calculation and the real world. Through quantum electrodynamics, physicists have been able to solidify the role of photons as the "smallest possible bundles of light" and to reveal their interactions with electrically charged particles such as electrons, in a mathematically complete, predictive, and convincing framework.
The success of quantum electrodynamics inspired other physicists in the 1960s and 1970s to try an analogous approach for developing a quantum-mechanical understanding of the weak, the strong, and the gravitational forces. For the weak and the strong forces, this proved to be an immensely fruitful line of attack. In analogy with quantum electrodynamics, physicists were able to construct quantum field theories for the strong and the weak forces, called quantum chromodynamics and quantum electroweak theory. "Quantum chromodynamics" is a more colorful name than the more logical "quantum strong dynamics," but it is just a name without any deeper meaning; on the other hand, the name "electroweak" does summarize an important milestone in our understanding of the forces of nature.
Through their Nobel Prize-winning work, Sheldon Glashow, Abdus Salam, and Steven Weinberg showed that the weak and electromagnetic forces are naturally united by their quantum field-theoretic description even though their manifestations seem to be utterly distinct in the world around us. After all, weak force fields diminish to almost vanishing strength on all but subatomic distance scales, whereas electromagnetic fields—visible light, radio and TV signals, X-rays—have an indisputable macroscopic presence. Nevertheless, Glashow, Salam, and Weinberg showed, in essence, that at high enough energy and temperature—such as occurred a mere fraction of a second after the big bang—electromagnetic and weak force fields dissolve into one another, take on indistinguishable characteristics, and are more accurately called electroweak fields. When the temperature drops, as it has done steadily since the big bang, the electromagnetic and weak forces crystallize out in a different manner from their common high-temperature form—through a process known as symmetry breaking that we will describe later—and therefore appear to be distinct in the cold universe we currently inhabit.
And so, if you are keeping score, by the 1970s physicists had developed a sensible and successful quantum-mechanical description of three of the four forces (strong, weak, electromagnetic) and had shown that two of the three (weak and electromagnetic) actually share a common origin (the electroweak force). During the past two decades, physicists have subjected this quantum-mechanical treatment of the three nongravitational forces—as they act among themselves and the matter particles introduced in Chapter 1—to an enormous amount of experimental scrutiny. The theory has met all such challenges with aplomb. Once experimentalists measure some 19 parameters (the masses of the particles in Table 1.1, their force charges as recorded in the table in endnote 1 to Chapter 1, the strengths of the three nongravitational forces in Table 1.2, as well as a few other numbers we need not discuss), and theorists input these numbers into the quantum field theories of the matter particles and the strong, weak, and electromagnetic forces, the subsequent predictions of the theory regarding the microcosmos agree spectacularly with experimental results. This is true up to the energies capable of pulverizing matter into bits as small as a billionth of a billionth of a meter, the current technological limit. For this reason, physicists call the theory of the three nongravitational forces and the three families of matter particles the standard theory, or (more often) the standard model of particle physics.
Messenger Particles
According to the standard model, just as the photon is the smallest constituent of an electromagnetic field, the strong and the weak force fields have smallest constituents as well. As we discussed briefly in Chapter 1, the smallest bundles of the strong force are known as gluons, and those of the weak force are known as weak gauge bosons (or more precisely, the W and Z bosons). The standard model instructs us to think of these force particles as having no internal structure—in this framework they are every bit as elementary as the particles in the three families of matter.
The photons, gluons, and weak gauge bosons provide the microscopic mechanism for transmitting the forces they constitute. For example, when one electrically charged particle repels another of like electric charge, you can think of it roughly in terms of each particle being surrounded by an electric field—a "cloud" or "mist" of "electric-essence"—and the force each particle feels arises from the repulsion between their respective force fields. The more precise microscopic description of how they repel each other, though, is somewhat different. An electromagnetic field is composed of a swarm of photons; the interaction between two charged particles actually arises from their "shooting" photons back and forth between themselves. In rough analogy to the way in which you can affect a fellow ice-skater's motion and your own by hurling a barrage of bowling balls at him or her, two electrically charged particles influence each other by exchanging these smallest bundles of light.
An important failing of the ice-skater analogy is that the exchange of bowling balls is always "repulsive"—it always drives the skaters apart. On the contrary, two oppositely charged particles also interact through the exchange of photons, although the resulting electromagnetic force is attractive. It's as if the photon is not so much the transmitter of the force per se, but rather the transmitter of a message of how the recipient must respond to the force in question. For like-charged particles, the photon carries the message "move apart," while for oppositely charged particles it carries the message "come together." For this reason the photon is sometimes referred to as the messenger particle for the electromagnetic force. Similarly, the gluons and weak gauge bosons are the messenger particles for the strong and weak nuclear forces. The strong force, which keeps quarks locked up inside of protons and neutrons, arises from individual quarks exchanging gluons. The gluons, so to speak, provide the "glue" that keeps these subatomic particles stuck together. The weak force, which is responsible for certain kinds of particle transmutations involved in radioactive decay, is mediated by the weak gauge bosons.
Gauge Symmetry
You may have realized that the odd man out in our discussion of the quantum theory of the forces of nature is gravity. Given the successful approach physicists have used with the other three forces, you might suggest that physicists seek a quantum field theory of the gravitational force—a theory in which the smallest bundle of a gravitational force field, the graviton, would be its messenger particle. At first sight, as we now note, this suggestion would appear to be particularly apt because the quantum field theory of the three nongravitational forces reveals that there is a tantalizing similarity between them and an aspect of the gravitational force we encountered in Chapter 3.
Recall that the gravitational force allows us to declare that all observers—regardless of their state of motion—are on absolutely equal footing. Even those whom we would normally think of as accelerating may claim to be at rest, since they can attribute the force they feel to their being immersed in a gravitational field. In this sense, gravity enforces the symmetry: it ensures the equal validity of all possible observational points of view, all possible frames of reference. The similarity with the strong, weak, and electromagnetic forces is that they too are all connected with enforcing symmetries, albeit ones that are significantly more abstract than the one associated with gravity.
To get a rough feel for these rather subtle symmetry principles, let's consider one important example. As we recorded in the table in endnote 1 of Chapter 1, each quark comes in three "colors" (fancifully called red, green, and blue, although these are merely labels and have no relation to color in the usual visual sense), which determine how it responds to the strong force in much the same way that its electric charge determines how it responds to the electromagnetic force. All the data that have been collected establish that there is a symmetry among the quarks in the sense that the interactions between any two like-colored quarks (red with red, green with green, or blue with blue) are all identical, and similarly, the interactions between any two unlike-colored quarks (red with green, green with blue, or blue with red) are also identical. In fact, the data support something even more striking. If the three colors—the three different strong charges—that a quark can carry were all shifted in a particular manner (roughly speaking, in our fanciful chromatic language, if red, green, and blue were shifted, for instance, to yellow, indigo, and violet), and even if the details of this shift were to change from moment to moment or from place to place, the interactions between the quarks would be, again, completely unchanged. For this reason, just as we say that a sphere exemplifies rotational symmetry because it looks the same regardless of how we rotate it around in our hands or how we shift the angle from which we view it, we say that the universe exemplifies strong force symmetry: Physics is unchanged by—it is completely insensitive to—these force-charge shifts. For historical reasons, physicists also say that the strong force symmetry is an example of a gauge symmetry.5
Here is the essential point. Just as the symmetry between all possible observational vantage points in general relativity requires the existence of the gravitational force, developments relying on work of Hermann Weyl in the 1920s and Chen-Ning Yang and Robert Mills in the 1950s showed that gauge symmetries require the existence of yet other forces. Much like a sensitive environmental-control system that keeps temperature, air pressure, and humidity in an area completely constant by compensating perfectly for any exterior influences, certain kinds of force fields, according to Yang and Mills, will provide perfect compensation for shifts in force charges, thereby keeping the physical interactions between the particles completely unchanged. For the case of the gauge symmetry associated with shifting quark-color charges, the required force is none other than the strong force itself. That is, without the strong force, physics would change under the kinds of shifts of color charges indicated above. This realization shows that, although the gravitational force and the strong force have vastly different properties (recall, for example, that gravity is far feebler than the strong force and operates over enormously larger distances), they do have a somewhat similar heritage: they are each required in order that the universe embody particular symmetries. Moreover, a similar discussion applies to the weak and electromagnetic forces, showing that their existence, too, is bound up with yet other gauge symmetries—the so-called weak and electromagnetic gauge symmetries. And hence, all four forces are directly associated with principles of symmetry.
This common feature of the four forces would seem to bode well for the suggestion made at the beginning of this section. Namely, in our effort to incorporate quantum mechanics into general relativity we should seek a quantum field theory of the gravitational force, much as physicists have discovered successful quantum field theories of the other three forces. Over the years, such reasoning has inspired a prodigious and distinguished group of physicists to follow this path vigorously, but the terrain has proven to be fraught with danger, and no one has succeeded in traversing it completely. Let's see why.
General Relativity vs. Quantum Mechanics
The usual realm of applicability of general relativity is that of large, astronomical distance scales. On such distances Einstein's theory implies that the absence of mass means that space is flat, as illustrated in Figure 3.3. In seeking to merge general relativity with quantum mechanics we must now change our focus sharply and examine the microscopic properties of space. We illustrate this in Figure 5.1 by zooming in and sequentially magnifying ever smaller regions of the spatial fabric. At first, as we zoom in, not much happens; as we see in the first three levels of magnification in Figure 5.1, the structure of space retains the same basic form. Reasoning from a purely classical standpoint, we would expect this placid and flat image of space to persist all the way to arbitrarily small length scales. But quantum mechanics changes this conclusion radically. Everything is subject to the quantum fluctuations inherent in the uncertainty principle—even the gravitational field. Although classical reasoning implies that empty space has zero gravitational field, quantum mechanics shows that on average it is zero, but that its actual value undulates up and down due to quantum fluctuations. Moreover, the uncertainty principle tells us that the size of the undulations of the gravitational field gets larger as we focus our attention on smaller regions of space. Quantum mechanics shows that nothing likes to be cornered; narrowing the spatial focus leads to ever larger undulations.
As gravitational fields are reflected by curvature, these quantum fluctuations manifest themselves as increasingly violent distortions of the surrounding space. We see the glimmers of such distortions emerging in the fourth level of magnification in Figure 5.1. By probing to even smaller distance scales, as we do in the fifth level of Figure 5.1, we see that the random quantum mechanical undulations in the gravitational field correspond to such severe warpings of space that it no longer resembles a gently curving geometrical object such as the rubber-membrane analogy used in our discussion in Chapter 3. Rather, it takes on the frothing, turbulent, twisted form illustrated in the uppermost part of the figure. John Wheeler coined the term quantum foam to describe the frenzy revealed by such an ultramicroscopic examination of space (and time)—it describes an unfamiliar arena of the universe in which the conventional notions of left and right, back and forth, up and down (and even of before and after) lose their meaning. It is on such short distance scales that we encounter the fundamental incompatibility between general relativity and quantum mechanics. The notion of a smooth spatial geometry, the central principle of general relativity, is destroyed by the violent fluctuations of the quantum world on short distance scales. On ultramicroscopic scales, the central feature of quantum mechanics—the uncertainty principle—is in direct conflict with the central feature of general relativity—the smooth geometrical model of space (and of spacetime).
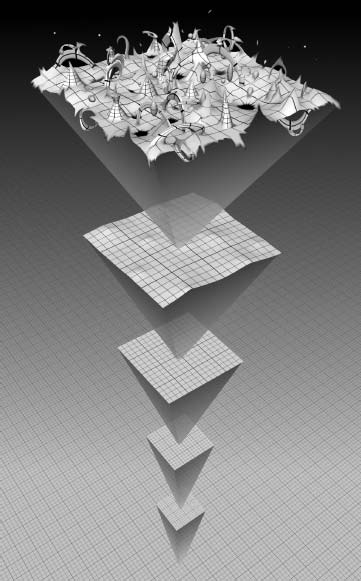
Figure 5.1 By sequentially magnifying a region of space, its ultramicroscopic properties can be probed. Attempts to merge general relativity and quantum mechanics run up against the violent quantum foam emerging at the highest level of magnification.
In practice, this conflict rears its head in a very concrete manner. Calculations that merge the equations of general relativity and those of quantum mechanics typically yield one and the same ridiculous answer: infinity. Like a sharp rap on the wrist from an old-time schoolteacher, an infinite answer is nature's way of telling us that we are doing something that is quite wrong.6 The equations of general relativity cannot handle the roiling frenzy of quantum foam.
Notice, however, that as we recede to more ordinary distances (following the sequence of drawings in Figure 5.1 in reverse), the random, violent small-scale undulations cancel each other out—in much the same way that, on average, our compulsive borrower's bank account shows no evidence of his compulsion—and the concept of a smooth geometry for the fabric of the universe once again becomes accurate. It's like what you experience when you look at a dot-matrix picture: From far away the dots that compose the picture blend together and create the impression of a smooth image whose variations in lightness seamlessly and gently change from one area to another. When you inspect the picture on finer distance scales you realize, however, that it markedly differs from its smooth, long-distance appearance. It is nothing but a collection of discrete dots, each quite separate from the others. But note that you become aware of the discrete nature of the picture only when you examine it on the smallest of scales; from far away it looks smooth. Similarly, the fabric of spacetime appears to be smooth except when examined with ultramicroscopic precision. This is why general relativity works on large enough distance (and time) scales—the scales relevant for many typical astronomical applications—but is rendered inconsistent on short distance (and time) scales. The central tenet of a smooth and gently curving geometry is justified in the large but breaks down due to quantum fluctuations when pushed to the small.
The basic principles of general relativity and quantum mechanics allow us to calculate the approximate distance scales below which one would have to shrink in order for the pernicious phenomenon of Figure 5.1 to become apparent. The smallness of Planck's constant—which governs the strength of quantum effects—and the intrinsic weakness of the gravitational force team up to yield a result called the Planck length, which is small almost beyond imagination: a millionth of a billionth of a billionth of a billionth of a centimeter (10-33 centimeter).7 The fifth level in Figure 5.1 thus schematically depicts the ultramicroscopic, sub-Planck length landscape of the universe. To get a sense of scale, if we were to magnify an atom to the size of the known universe, the Planck length would barely expand to the height of an average tree.
And so we see that the incompatability between general relativity and quantum mechanics becomes apparent only in a rather esoteric realm of the universe. For this reason you might well ask whether it's worth worrying about. In fact, the physics community does not speak with a unified voice when addressing this issue. There are those physicists who are willing to note the problem, but happily go about using quantum mechanics and general relativity for problems whose typical lengths far exceed the Planck length, as their research requires. There are other physicists, however, who are deeply unsettled by the fact that the two foundational pillars of physics as we know it are at their core fundamentally incompatible, regardless of the ultramicroscopic distances that must be probed to expose the problem. The incompatibility, they argue, points to an essential flaw in our understanding of the physical universe. This opinion rests on an unprovable but profoundly felt view that the universe, if understood at its deepest and most elementary level, can be described by a logically sound theory whose parts are harmoniously united. And surely, regardless of how central this incompatibility is to their own research, most physicists find it hard to believe that, at rock bottom, our deepest theoretical understanding of the universe will be composed of a mathematically inconsistent patchwork of two powerful yet conflicting explanatory frameworks.
Physicists have made numerous attempts at modifying either general relativity or quantum mechanics in some manner so as to avoid the conflict, but the attempts, although often bold and ingenious, have met with failure after failure.
That is, until the discovery of superstring theory.8